Student Learning Objectives
Lessons / Lecture Notes
Important Equations
Example Problems
Applets and Animations
Student Learning Objectives
Lessons / Lecture Notes
The Physics Classroom (conceptual)
PY105 Notes from Boston University (algebra-based):
Physics 2C notes from Dr. Bobby W.S. Lau (algebra-based)
HyperPhysics (calculus-based)
Physics 4C notes from Dr. Bobby W.S. Lau (calculus-based)
Important Equations (for algebra-based Physics)
Example Problems
Example Problems for algebra-based physics (from College Physics 2nd Edition by Knight, Jones, and Field):
Example Problems (Waves and Superposition)
Solutions to Example Problems (Waves and Superposition)
Example Problems for calculus-based physics (from Physics for Scientists and Engineers 4th Edition by Knight):
Example Problems (Traveling Waves and Superposition)
Solutions to Example Problems (Traveling Waves and Superposition)
| Interference | 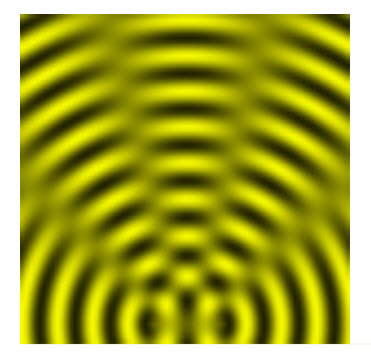 |
A very nice animation showing the interference patterns from two wave sources. The user can adjust the wavelength, source separation, and phase of the wave sources. |
| Wave Interference | 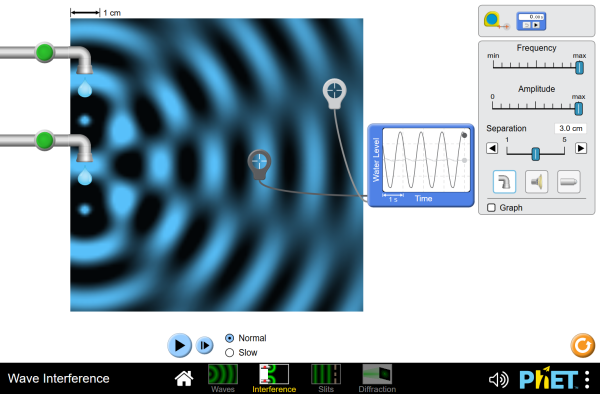 |
Make waves with a dripping faucet, audio speaker, or laser! Add a second source to create an interference pattern. Put up a barrier to explore single-slit diffraction and double-slit interference. Experiment with diffraction through elliptical, rectangular, or irregular apertures. |
| Ripple Tank | 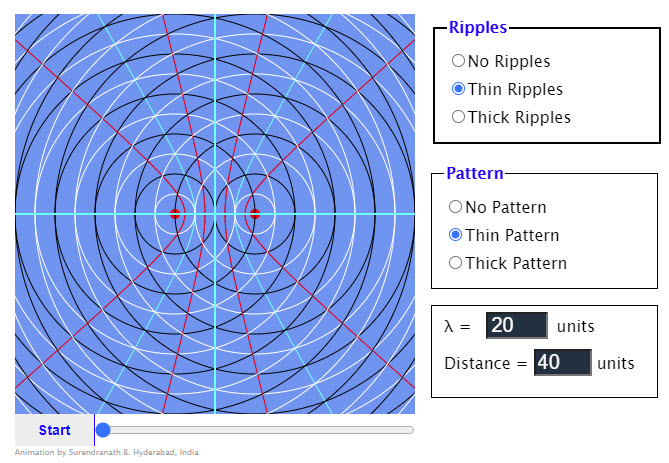 |
Animation shows interference of waves from two point sources. The white circles can be imaginged to be crests and the black circles can be imagined to be troughs. The cyan line passes through points where interference is constructive . On this line you can see crest and crest or trough and trough combining. The red line passes through points where interference is destructive. On this line crest and trough combine. You can change the wavelength of the waves and the distance between the sources using scrollbars. There are options for viewing thick or thin lines to suit individual taste and an option to turn the pattern off to have an uncluttered view of the ripples. The option of no ripples would allow only the lines of constuctive and destuctive interference to be seen. Experiment with distances which are multiples of a wavelength and see what happens. Also with the animation stopped, change the distance between the sources and observe cyan and the red curves. Turn off the ripples and change the distance between the sources are the wavelength and observe the pattern of the curves.
|
| Ripple Tank | 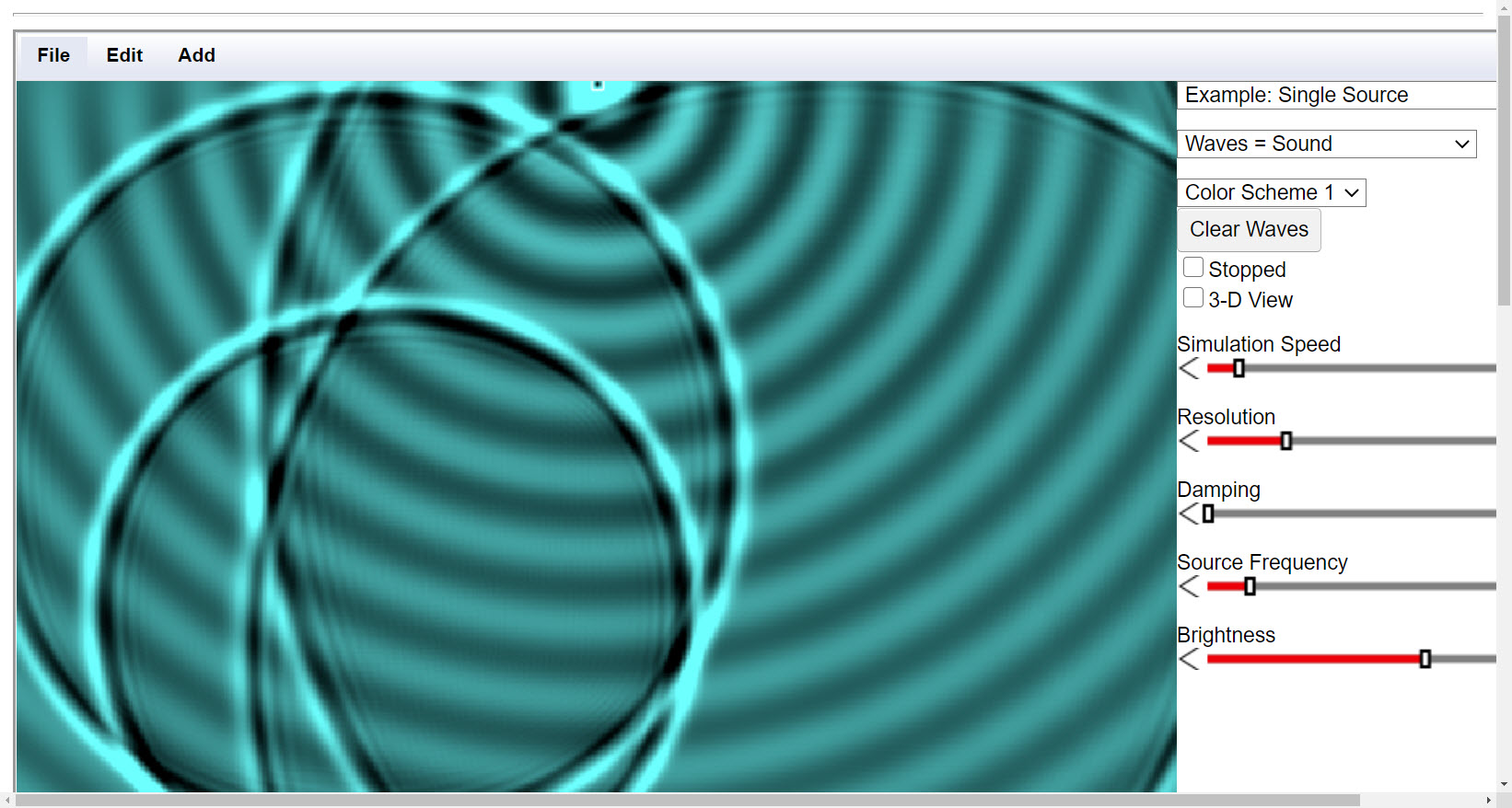 |
Great animation showing a ripple tank with numerous very cool options. I highly recommend exploring the many different setups. |
| Reflection and Transmission | This is a simulation of a wave traveling through one medium. If the wave encounters a fixed end, the wave is reflected upside down. If the wave encounters a free end, the wave is reflected upright. This is relevant for light, when it encounters an interface between two boundaries. The light string is analogous to a low-n (fast) medium, and the heavy string is analogous to a high-n (slow) medium. |
|
| Pulse Reflection at String End | 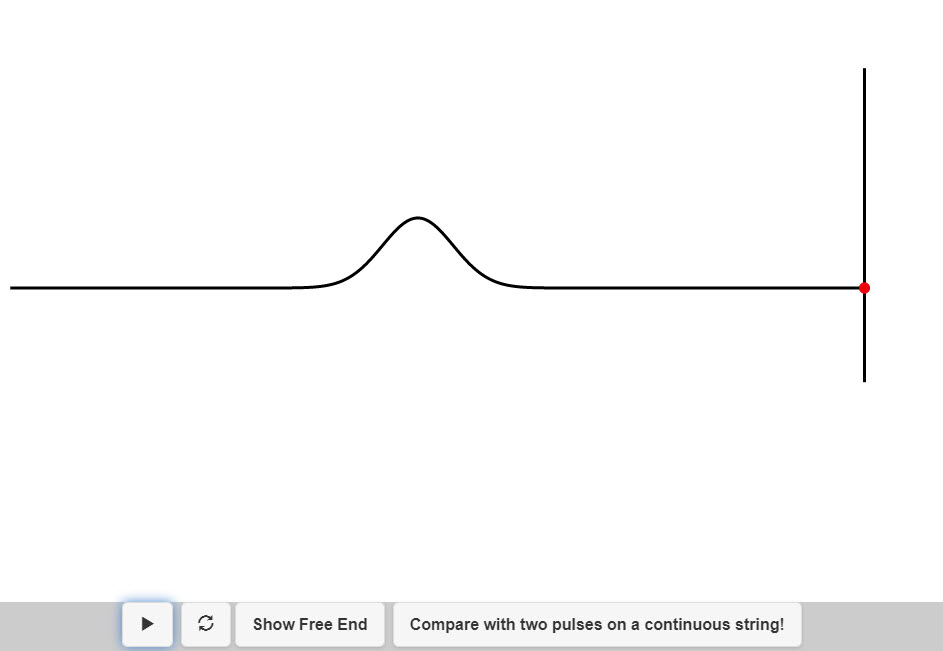 |
Another animation showing the reflection of a wave on a string from either a fixed end or a free end. |
| Wave Pulse |  |
In this useful applet, you can investigate the reflection and transmission of waves on a string at an interface between strings of different linear mass densities. |
| Transverse Wave: Reflection and Transmission | A pulse is shown to be travelling right which undergoes a reflection or is reflected and transmitted across a boundary of two ropes. You can see the incident, reflected and transmitted components during the process. The white pulse is the incident pulse and the red pulse is the reflected pulse You should be able to notice the change in wavelength and amplitude during reflection, transmission across a boundary. |
|
| Reflections | This applet shows the reflections of a wave from either a fixed or free end. | |
| Constructive and Destructive Interference |
This nice animation show the contructive and destructive interference of two waves traveling in opposite directions. |
|
| Constructive and Destructive Interference | 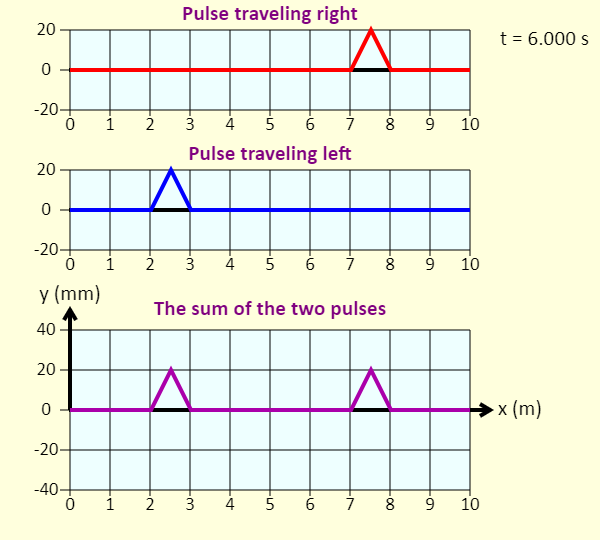 |
Another nice animation showing the contructive and destructive interference of two waves traveling in opposite directions. |
| Transverse Wave - Addition | The disturbance shown in red color is due to a source somewhere beyond the left end. The disturbance shown in white color is due to a source somewhere beyond the right end. The resultant disturbance is shown in yellow color. | |
| Transverse Wave - Addition |
The disturbance shown in red color is due to a source somewhere beyond the left end. The disturbance shown in blue color is due to a source somewhere beyond the right end. The resultant disturbance is shown in yellow color. |
|
| Standing Wave Explanation |
Outstanding animation showing the formation of a standing wave by the superposition of the incident wave and the reflected wave. |
|
| A Standing Wave on a String | 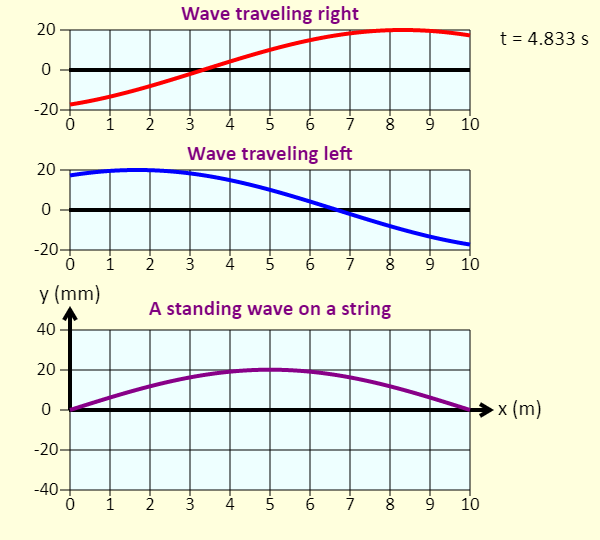 |
Nice animation showing the formation of a standing wave by the superposition of the incident wave and the reflected wave. |
| Generating Standing Waves on String (Resonance) |
See how many different standing waves you can produce by adjusting the frequency of the wave generator. |
|
| Wave on a String |  |
Explore the wonderful world of waves! Even observe a string vibrate in slow motion. Wiggle the end of the string and make waves, or adjust the frequency and amplitude of an oscillator. |
| A longitudinal standing wave |
Nice animation showing the different stading waves that can be set up in a pipe open at one end or at both ends. |
|
| Standing Longitudinal Waves | This HTML5 app demonstrates the harmonics of the air in a tube as an example of standing longitudinal waves. It illustrates the movement of the molecules in the air during such an oscillation. (Obviously the particles in reality move much shorter distances, and the real movement is very quick.) The nodes, i.e. the places where the particles don't move, are marked with "N". "A" means an antinode, i.e. a place where the particles oscillate with maximal amplitude. Note that at an open end of the tube there is always an antinode, at a closed end, however, a node! You can select the form of the tube by using the appropriate radio buttons ("both sides open", "one side open" and "both sides closed"). It is possible to switch to the next harmonic by pressing the button "Lower" respectively "Higher". The app shows the harmonics up to the fifth upper oscillation. If you write a new value for the length of the tube into the textfield and press the "Enter" key, the app will calculate wavelength and frequency. The speed of sound was presupposed as 343.5 m/s, corresponding to a temperature of 20°C. The influence of the tube's diameter is neglected. |
|
| Standing Waves on a Square Membrane | 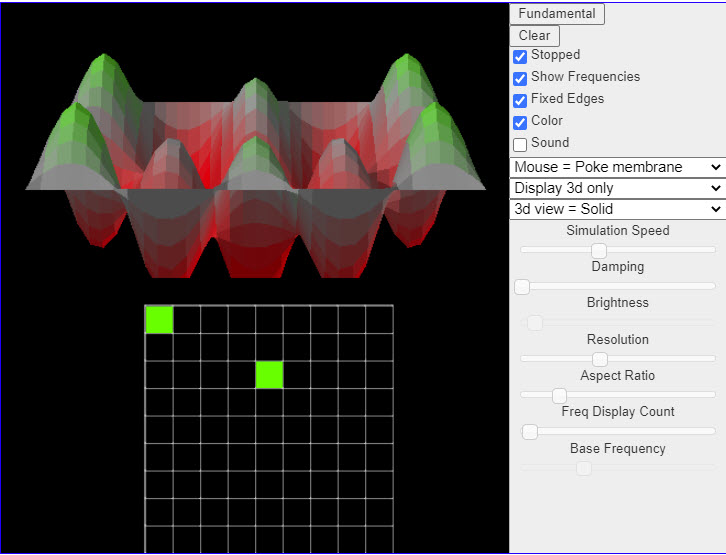 |
Very cool applet showing the formation of standing waves on a square membrane. |
| Standing Waves on a Circular Membrane |  |
Very cool applet showing the formation of standing waves on a circular membrane. |
| Pendulum Waves | 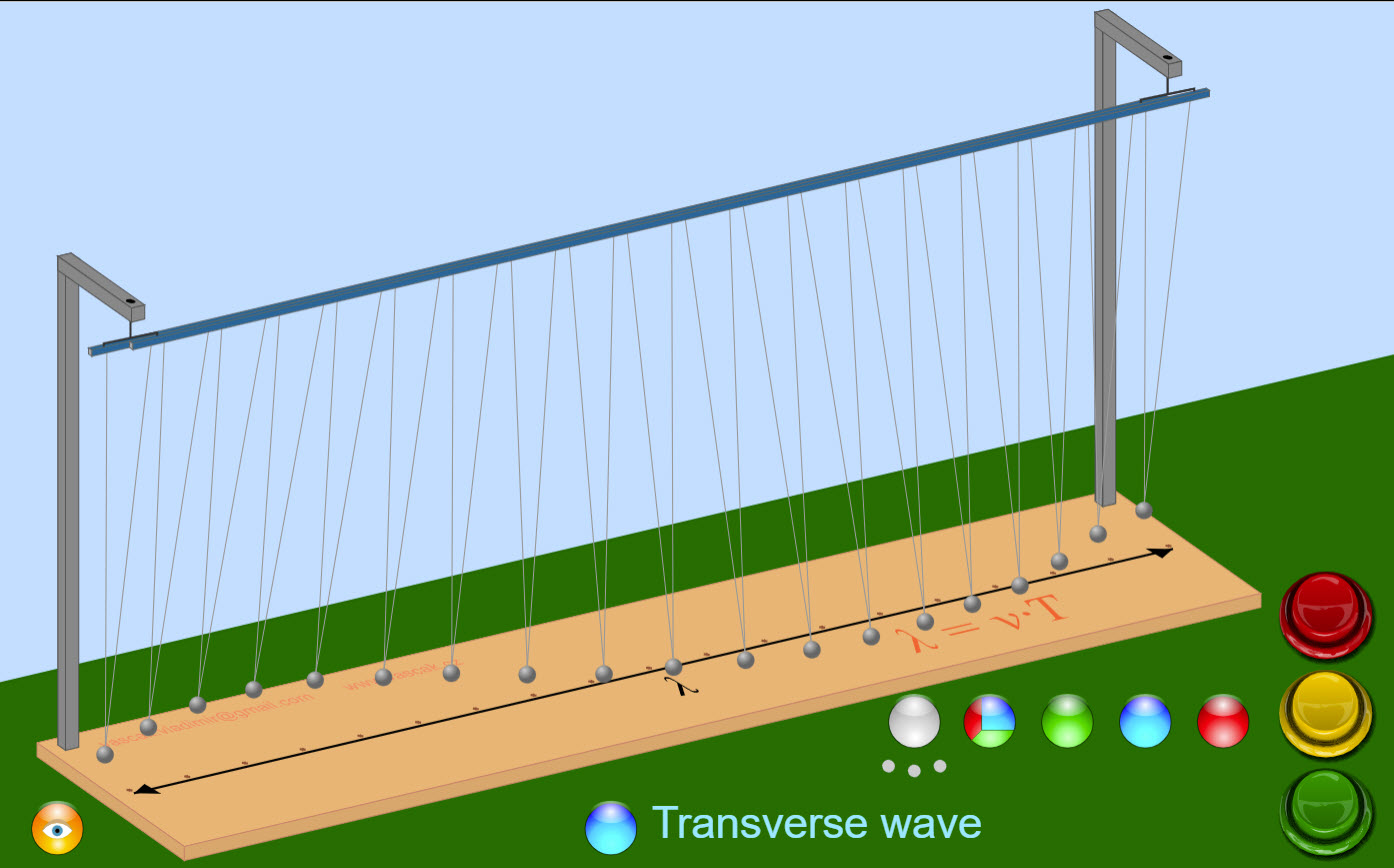 |
A very cool animation showing pendulum waves from different perspectives. |
| Resonance |
For advanced undergraduate students: Observe resonance in a collection of driven, damped harmonic oscillators. Vary the driving frequency and amplitude, the damping constant, and the mass and spring constant of each resonator. Notice the long-lived transients when damping is small, and observe the phase change for resonators above and below resonance. |
|
| Measuring Speed of Sound | Nice animatuion showing how to measure the speed of sound using standing waves in an air column. | |
| Beats | 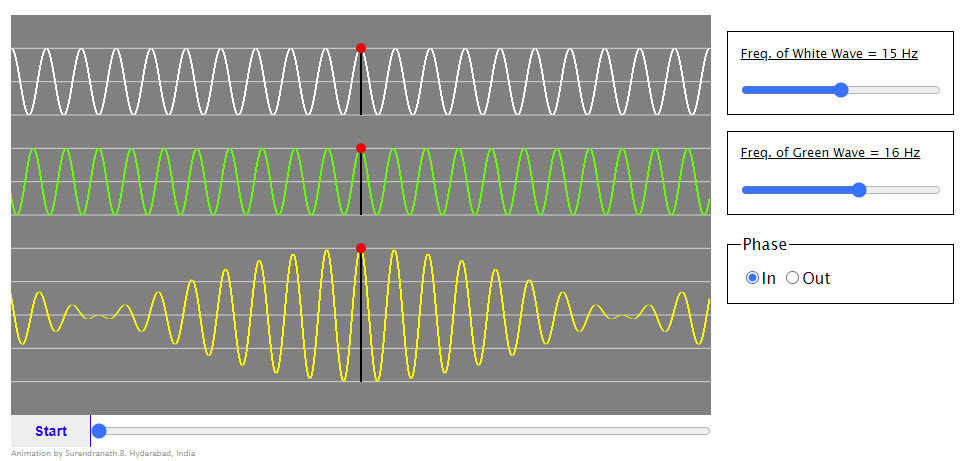 |
Simple animation where you can vary the frequencies of two sound wave and see the resulting beats. The sound waves can also be placed out of phase. |
| Beats | 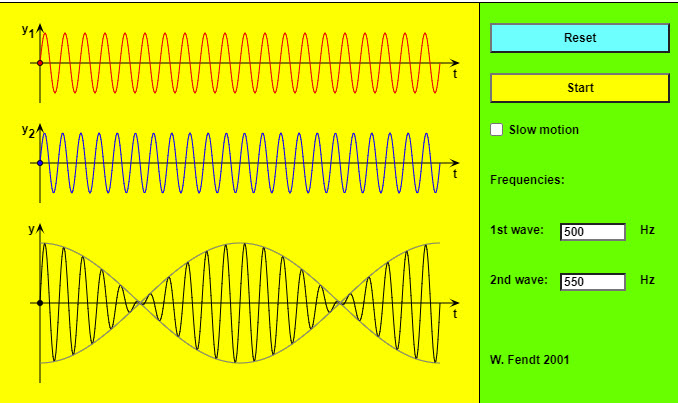 |
Nice animation showing the formation of beats. |
| Forming Beats |  |
Simple yet effective. Play two different sound waves at the same time (or individually) and hear the resulting beats. |
| Dispersion | 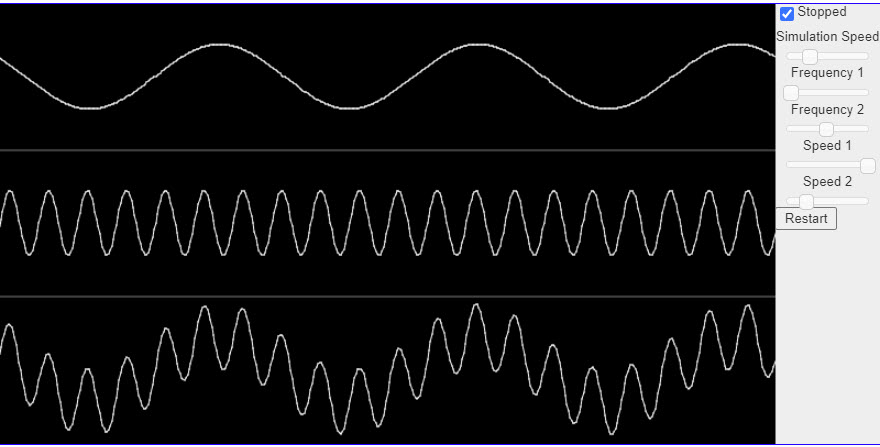 |
This java applet demonstrates dispersion, which occurs when the speed of a wave depends on frequency. This example shows two single-frequency waves on top, followed by the sum of the two on the bottom. In this case, the lower-frequency wave has a higher speed (phase velocity), which causes the sum to change shape as it propagates through space. |